Reynolds number effects: For an airfoil Reynolds number is usually defined as
This laboratory is designed to
You will be able to use this technique in a small smoke tunnel to study
a number of flows, including particularly the flow past a stalled airfoil.
Generating a flow in a wind tunnel that accurately models the flow over
a real vehicle or vehicle component can be a lot harder than just having
a model the right shape. During the course of this laboratory you will
be able to investigate some of these problems. In particular you will be
able to examine:
Reynolds number effects: For an airfoil Reynolds number
is usually defined as ![]() where
where ![]() is the free stream velocity,
c
the wing chord and
is the free stream velocity,
c
the wing chord and![]() the kinematic viscosity.
It represents the typical ratio of the scale of inertial forces to that
of viscous forces in the flow. Most engineering devices operate at large
Reynolds numbers. A airplane wing with a chord length of 7ft, flying at
150 km/hr at sea level has a Reynolds number of 5,700,000. A hydroplane
1m in chord traveling at 35 knots (18 m/s) has a Reynolds number of 19,400,000.
Reproducing such Reynolds numbers in a wind tunnel with a small model (say
0.25m chord) is usually not possible. Much wind tunnel work therefore relies
on the assumption that flows remain largely unaltered by increase in Reynolds
number. This is not always the case.
the kinematic viscosity.
It represents the typical ratio of the scale of inertial forces to that
of viscous forces in the flow. Most engineering devices operate at large
Reynolds numbers. A airplane wing with a chord length of 7ft, flying at
150 km/hr at sea level has a Reynolds number of 5,700,000. A hydroplane
1m in chord traveling at 35 knots (18 m/s) has a Reynolds number of 19,400,000.
Reproducing such Reynolds numbers in a wind tunnel with a small model (say
0.25m chord) is usually not possible. Much wind tunnel work therefore relies
on the assumption that flows remain largely unaltered by increase in Reynolds
number. This is not always the case.
Blockage effects: Blockage may occur as a result of the solid walls of a test section constraining the flow as it moves around a model. This constraint, which does not occur in free flight, increases the velocity of the flow as it passes the model, altering the flow pattern and characteristics. In the case of an airfoil, solid blockage tends to make the flow over an airfoil stall earlier than it otherwise would. This is because boundary layer separation occurs when in regions where the flow is decelerating, i.e. there is an adverse pressure gradient. The stronger the gradient the sooner separation will occur. Blockage increases the magnitude of the deceleration and thus the adverse pressure gradient produced by an airfoil. Interestingly, the reverse effect occurs in an open-jet wind tunnel. Here the wind-tunnel 'wall' is formed by the edge of the jet, and thus it can be deformed by the flow over the model. This deformation tends to reduce the magnitude of the accelerations and declarations experienced over the model.
A. Instrumentation for measuring the properties of the air.
The wind tunnel you will be given to use in this experiment
uses the laboratory atmosphere as the working fluid. The properties of the
air in the lab vary depending on the weather so it is important that at
some stage in your experiment that you measure them, so you know what fluid
you are working with. From the point of view of the dynamics of the air,
the important properties are its density and viscosity (think of Bernoulli's
equation and the Reynolds number).
Rather than measuring density directly, it is best obtained by measuring
pressure and temperature and then using the equation of state for a perfect
gas. An aneroid barometer for measuring atmospheric pressure is provided
on the side of the open-jet wind tunnel control panel which is in the same lab
as your experiment (figure
1 ). A digital thermometer for measuring atmospheric temperature is
located on the side of the open-jet tunnel next to the test section (also
shown in
figure 1 ). Pressure is read in milliBar (1
milliBar=100Pa). Temperature is read in degrees Celsius or Fahrenheit,
depending on the thermometer setting. The gas constant R in the
equation of state for a perfect gas (p =![]() RT) is 287 J/kg/K.
RT) is 287 J/kg/K.
The temperature can also be used to infer the dynamic viscosity of the air using Sutherland's relation. For SI units,
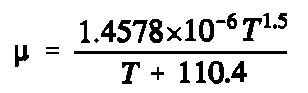
B. Smoke flow visualization wind tunnel and equipment
A small vertical smoke tunnel (figure 2 ) will be
at your disposal. The tunnel is an open-circuit design, powered by a small
variable speed electric fan. The top mounted fan pulls air from the room
through a turbulence screen into the lower entrance, which measures 35.6
cm x 17.8 cm. From the entrance the tunnel converges to the test section,
which measures 10.2 cm x 17.8 cm in cross-section and is 25.4 cm long.
Downstream from the test section, the tunnel converges to a 12.7 cm diameter
circle at the fan. The speed of the flow in the test section is controlled
using the dial located at the bottom right corner of the facility. The
calibration that relates the flow speed in the test section to the dial
setting is shown in figure
3 . The uncertainty of this calibration is not known, but it is probably no
better than 10%.
The "smoke", which is vaporized kerosene, is produced using a Preston-Sweeting mist generator (figure 4 ). The resulting vapor is piped via a black rubber hose into a strut located directly upstream from the test section. A series of equally spaced holes in the trailing edge of the smoke strut introduce smoke filaments into the flow. During operation of the smoke tunnel it may be necessary to give a sharp squeeze to the black rubber bulb attached to the end of the strut. This will clear anything that may be blocking the smoke holes. In addition, the black rubber hose connecting the smoke generator to the strut should be drained periodically or whenever the vapor flow is poor. To drain the hose, remove the end connected to the smoke generator, hold it towards the floor and give it a few shakes. Take precautions not to shake the kerosene on your pants leg, as this is easy to do.
The tunnel comes with some additional supplies and equipment including,
A. Getting familiar with the equipment
The following procedures are designed to help you
get a feel for the smoke tunnel, its models and peripheral equipment. It is important that
everybody get a hands on feel of how to use the apparatus and what its
capabilities and problems are. Feel free to play with the apparatus at
this stage, but don't forget to record your impressions in the logbook.
Goal 2. Design, conduct and document a sequence of tests to take
careful flow visualization pictures of a range of flows you have discussed
previously in AOE 3014 at a fixed Reynolds number, and to assess how wind
tunnel effects may have influenced your results. (This would make a nice
report later, where you can contrast what the pictures show with your expectations
from the earlier course, referencing the text book.)
Suggestions: Don't forget your lists of things to measure in
a real test - careful documentation of each flow will greatly enhance the
value of your results. Choose a Reynolds number where you get good pictures.
See suggestions above on wind tunnel effects. Be sure to record your impressions
of each flow in the electronic log book as you see the flows, what was
expected and what was not, you can see much more now than you will be able
to observe later in a photo.
Analysis suggestions for later. Compute the Reynolds number and
its uncertainty. Label pictures to indicate
the significant features of each flow, and the significant imperfections
of the wind tunnel flow - this will support any discussion you want to
make in your report. Make or copy (give a proper reference) figures showing
the form of these flows as you were introduced to them in AOE 3014. Consider
a quantitative comparison with theory. E.g. you could compare streamlines
in the flow past the circular cylinder with actual calculations based on
2D potential flow theory. Show the theoretical and experimental results
side-by-side, or over top of each other. Include details of the theoretical
calculation in your report.
Goal 3. Choose a model flow. Design, conduct and document a sequence
of tests to examine the effect of Reynolds number upon that model flow,
and assess the extent to which wind tunnel effects may have influenced
your results.
Suggestions: You may wish to run a preliminary test to see if
there is a model flow that has a greater Reynolds number dependence, as
that would be easier to study. Look at the lists you generated above. Having
the camera fixed on the tripod may be critical here in usefully comparing
photos taken at different speeds, and without the model (at different speeds),
as effects may be small. Try plotting significant features of the flow,
e.g. separation location, tip vortex position, vs. Reynolds number as you
go. If there are strange points on the curve, consider going back and re-measuring.
Analysis suggestions for later. Compute the Reynolds numbers
and their uncertainty. Add error bars to your
plots showing the locations of significant features vs. Reynolds number.
For assessing Reynolds number effects associated with the wind tunnel alone,
you could measure off streamline positions at the bottom of the wind tunnel
window, with and without model, and plot them against Reynolds number.
Labeling pictures to indicate the significant features of each flow, and
the significant imperfections of the wind tunnel flow will support the
discussion you make in your report. Don't forget that you can also discuss
the effects of Reynolds number upon the flow visualization technique itself
- e.g. you could measure of the width of a representative smoke stream
at the top of the test section window, with no model, vs. Reynolds number.
Plotting this would then show the change in diffusion rate of the smoke
as speed is increased.
Before starting your report please read all of appendix 1 . Check out the report grade sheet for this experiment, available in appendix 1..
Title page
As detailed in appendix 1.
Introduction
State logical objectives that best fit how your particular investigation
turned out and what you actually discovered and learned in this experiment (no
points for recycling the lab manual objectives). Then summarize what was done to
achieve them. Follow this with a background to the technical area of the test and/or
the techniques (e.g. the fluid dynamics and/or the experimental techniques you
are using). This material can be drawn from the manual (no copying), classes,
textbooks from prior courses, references cited in this chapter or even better,
other sources you have tracked down yourself. Finish with a summary of the layout of the rest of the report.
Apparatus and Instrumentation
It is probably easiest
to begin by describing the smoke tunnel itself. Include a diagram or labeled
photo of the tunnel. Describe the figure in the text of the report including
all the details that may be important to the flow it produces (e.g. open
circuit, contraction ratio, dimensions and shape of the test section, type
of vapor used, method of vapor production, method used to introduce vapor
to the flow, location of smoke strut, flow speed uncertainty etc.). Next describe the model(s)
you used. Diagrams or photos will be needed. Give the model dimensions
(span, chord, diameter, airfoil shape designation) and location(s) that
the models were placed in the test section (with the airfoil you should
also include the chordwise location about which it was rotated to angle
of attack). Don't forget to mention imperfections in the models and any
uncertainties. Now mention
the techniques used to make the measurements. Include in particular the
digital camera, tripod and location. If you measured positions directly
off the wind tunnel window, talk about how that was done (and the accuracy).
Mention the thermometer and barometer, their accuracies, and what they
were used for.
Results and Discussion
Before writing the results and discussion make sure all your results
are analyzed and plotted, your photographs are properly annotated and labeled.
Make sure your plots are formatted correctly - default Excel plotting format
is not acceptable, see appendix
1 .
A good way of writing this section may be to tie each set of tests and results to your objectives stated in the introduction. (If you find it hard to do this, try changing your objectives!) For example, you might begin with "Photographs of the smoke flow visualization were made with the airfoil model at between -15 and 15 degrees in order to define its stall behavior as a function of angle of attack. Figure ?? shows photographs of the flow at 3 degree increments in angle of attack. Table ?? and figure ?? show measurements of the chordwise location of stall at 1 degree increments in angle of attack. Included in figure ?? are error bars showing the uncertainty in the indicated stall locations...". Before you can really talk much in detail about variations seen with whatever parameter you are studying you will probably need to describe one case in detail, e.g. "Figure ??, which shows the flow pattern at 6 degrees angle of attack is typical. Flow approaching the airfoil stagnation point is deflected upwards and .... The streamlines passing over the top surface of the ..." Then talk about the variations, introduce your plots describe their axes, the symbols used and then discuss what they show". Don't forget the plots that show wind tunnel effects, e.g. "Figures ?? to ?? show flow through the test section for the same conditions as figure ?? but with the model removed. Comparing the figures measured at corresponding Reynolds number, some of the change in position of the streamlines with Reynolds number can be seen to be a inherent effect of the wind tunnel, and this would appear to bring into question...". Also remember to include any uncertainty estimates in derived results (such as for Reynolds number) here. You should reference a table (copied out of your Excel file) or appendix containing the uncertainty calculation.
Make sure your results and discussion include (and justify) the conclusions you want to make and that those conclusions connect with your objectives.
Conclusions
Begin with a brief description of what was done. Then a sequence of
single sentence numbered conclusions that express what was learned. Your
conclusions should mesh with the objectives stated in the introduction
(if not, change the objectives) and should be already stated (although
perhaps not as succinctly) in the Results and Discussion.
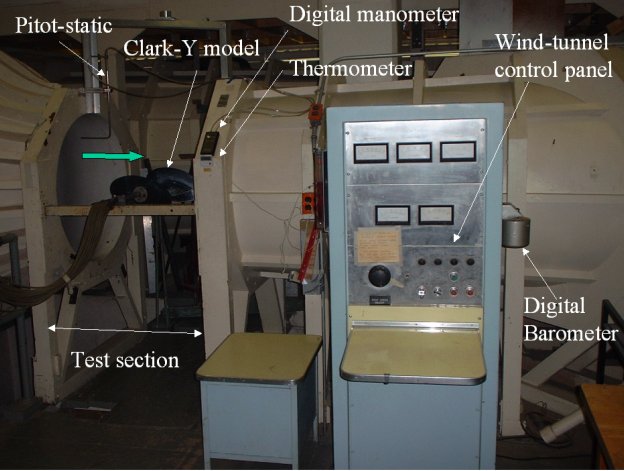
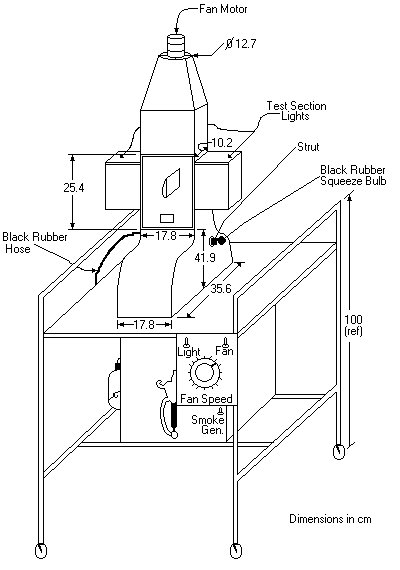
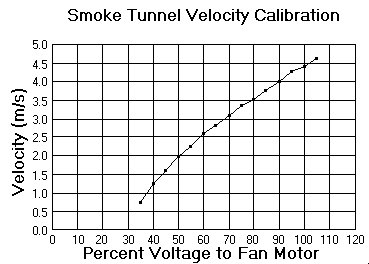
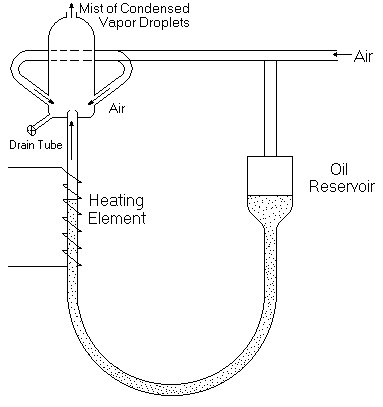
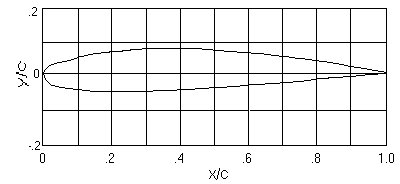

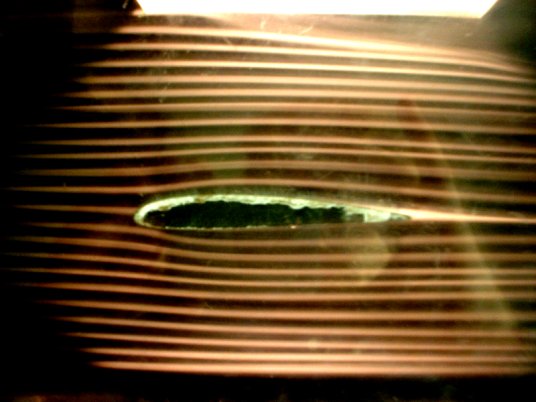
Figure 7. Sample smoke flow visualization for the airfoil model.